2015 September 12

Fun fact: the sum of $1/(ab)^2$ over all relatively prime pairs of positive integers $a$ , $b$ is rational. This is not true if you remove the “relatively prime” restriction.

Short proof… that sum equals

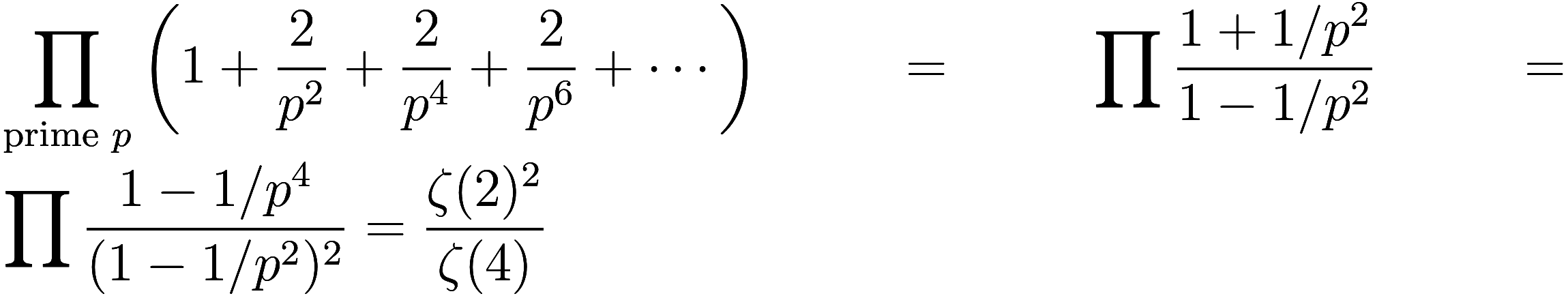
$\prod_{\text{prime } p} \left(1 + \frac 2{p^2} + \frac 2{p^4} + \frac 2{p^6} + \cdots\right) = \prod \frac {1 + 1 / p^2}{1 - 1 / p^2} = \prod \frac {1 - 1 / p^4}{(1 - 1 / p^2)^2} = \frac {\zeta(2)^...$

where $\zeta$ is the Riemann zeta function. Since $\zeta(k)$ is equal to $\pi^k$ times a rational when $k$ is positive even, the $\pi$ s cancel and the result is rational, in fact $5/2$ . If you remove the restriction you get $\zeta(2)^2 = \pi^4 / 36$ which is irrational. You can make other combinations of $\zeta$ s so that the $\pi$ s cancel to get other rational infinite summations, for example the sum of $1/(abc)^2$ where $a$ is squarefree and divides $b$ should give $\zeta(2)^2 \zeta(4) / \zeta(8) = 35 / 12$ , and in both examples replacing the exponent 2 by any even integer also works.

Follow RSS/Atom feed for updates.